Structure in Chemistry
Molecular Orbital Theory
MO2a. Wave Behaviour
In the early 20th Century, our understanding of light and matter was progressing very quickly, but some things still didn't make sense to people. Things really started to click when a number of physicists started thinking about things in a different way. One of the revolutionary things they did was to treat seriously, with mathematical equations, the wave nature of light and matter.
In the case of light, the idea actually dates back to the mid-17th Century. Christiaan Huygens, a Dutch clockmaker and astronomer, believed that light traveled in waves because he could see evidence of interference patterns in light, much like the way ripples on a pond can interfere with each other to produce new patterns. Other scientists, such as Newton, really thought light was made of small particles. In a sense, both were right, and we now think of particles of light, or photons, as having some of the properties of waves.
What do we know about waves? Waves on the surface of a lake or an ocean are good examples. You probably took a detailed look at them around 6th grade, although you may have forgotten some of the details. So, let's take another look.
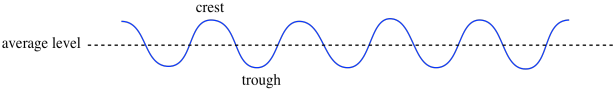
Maybe you have stood beside a wave tank at a science museum and seen something that looks like this. The surface of the water undulates up and down. There are crests where the water mounds up in hills and troughs where the water slips away into valleys. Somewhere in between, there is an average water level, where the surface would settle if things were calm and there were no waves at all.
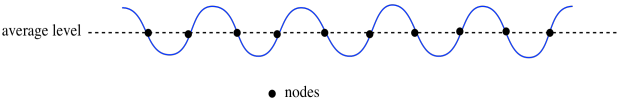
We can also think of waves as having null points or nodes; those are the positions halfway in between the crest and the trough.
Max Planck and Albert Einstein each took a closer look at that idea in the early 1900's when they were trying to determine the relationships between electrons in materials and their interaction with electromagnetic radiation. Electromagnetic radiation includes things like ultraviolet and visible light.
If we are standing at the seaside, one of the first things we notice about the waves is how tall they are. Maybe it's a really calm day and the waves are almost flat. Maybe it's a really rough day and the waves are really tall. The feature of waves that you are noticing is called the amplitude. That's the height of the wave from the average water lavel to the crest, or, conversely, the depth down to the trough. In the macroscopic world, the amplitude of the waves is really important. You need to know whether it's OK to stand and watch the waves, or whether it's getting to rough and you should seek higher ground. If you aren't careful, a really high amplitude wave could come crashing down on you.
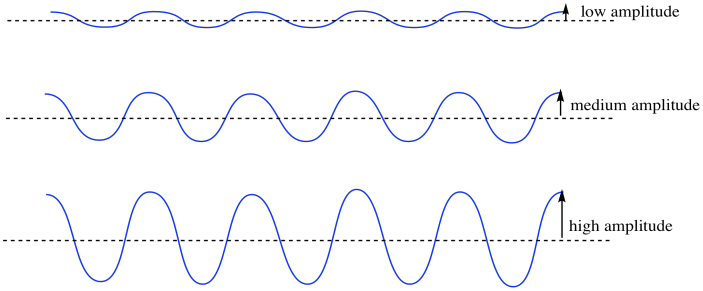
Waves on the ocean can have high amplitude or low amplitude, and there is a physical consequence. Sound waves can also have high amplitude (those are the loud ones) or low amplitude (those are the quiet ones). Of course, if the amplitude were really, really low, we would almost have no wave at all (when that person is whispering a secret but you can't even hear them).
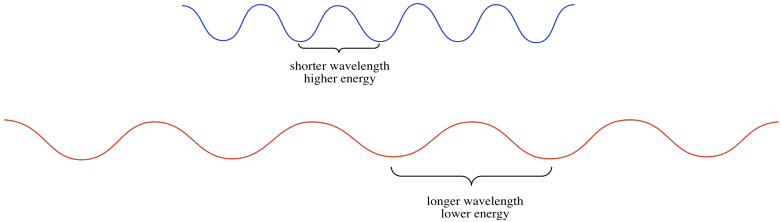
In the nanoscale world, the world of photons and electrons, the amplitude of a wave is a much more subtle thing. It's related to probability. That isn't our first consideration at the moment. What's much more important for our discussion right now is the wavelength. The wavelength is just the distance from one crest to the next, or from one trough to the next.
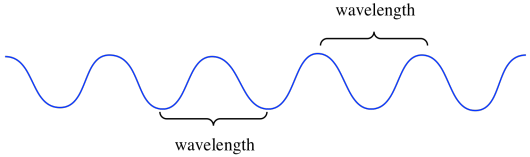
The wavelength is really important because, according to Planck and Einstein's analysis of experimental data, it's what determines the energy of an individual photon, or the energy of an individual electron. The shorter the wavelength of a photon or electron, the higher its energy. The longer the wavelength of a photon or electron, the lower its energy. This relationship is an example of inverse proportionality: when one gets bigger, the other gets smaller.
There is a complementary attribute of waves, and that is frequency. We can think of frequency as how often a wave crests. Alternatively, if we picture an electromagnetic wave passing a stationary object, the frequency would describe how oftena crest passes the object. (All light travels at the same speed, about 3 x 108 m s-1, so we don't have to worry about that being a factor.)
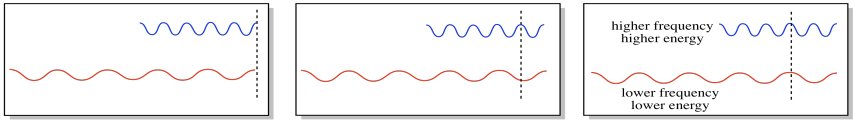
Frequency is inversely proportional to wavelength: the longer the wavelength, the lower the frequency. Consequently, frequency is directly proportional to the energy of the photon or electron. The higher the frequency, the higher the energy.
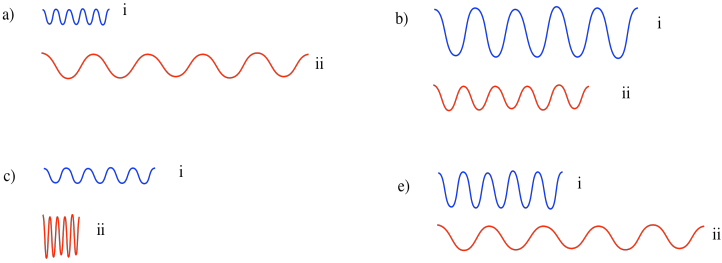
Problem MO2a.1.
Between each pair, which wave has greater energy?
Let's return to Christiaan Huygens' original observation of interference patterns in light. What does that mean?
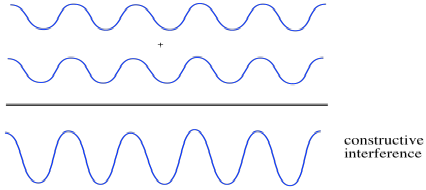
Suppose two waves come together. We'll think about two different cases. In the first case, the two waves are in phase with each other. That means that they both crest at the same time and their troughs are always aligned, too. What happens then? You can imagine the crests will just build on each other, getting taller, and the troughs will fall into each other, getting deeper. The amplitude of the waves, together, increases. This is constructive interference.
Now suppose that the two waves are out of phase. When one crests, the other bottoms out. In that case, the crest of one wave falls into the trough of the other. Everything levels out. This is destructive interference.
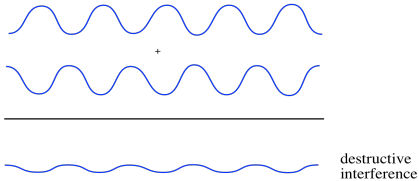
Constructive interference and destructive interference also occurs with waves on the water. That leads to lots of ripple patterns if you toss two stones into a still pond. Photons can also produce those kinds of patterns, and so can electrons.
This site was written by Chris P. Schaller, Ph.D., College of Saint Benedict / Saint John's University (retired) with other authors as noted on individual pages. It is freely available for educational use.
Structure & Reactivity in Organic, Biological and Inorganic Chemistry
by Chris Schaller is licensed under a
Creative Commons Attribution-NonCommercial 3.0 Unported License.
Send corrections to cschaller@csbsju.edu
Navigation: