Beginning Equilibrium Concepts

Reversible/Irreversible Reactions, Extent of Reactions, Equilibrium: Consider a hypothetical reversible reaction in which you start with some reactants, A and B, each at a 1 M concentration (1 mol of each/L solution). but no products, P and Q. For ease assume that the total volume of solution is 1 L, so that we start with 1 mol each of A and B.. At time t=0, the concentration of products is 0. The reaction can be written as:

As time progresses, the amounts or concentrations of A and B decrease as the amounts or concentrations of products P and Q increase. At some time, no further changes occur in the amount or concentrations of remaining reactants or products. At this point the reaction is in equilibrium, a term used often in our common vocabulary to denote a system that isn't changing.).

Most of the reactions that we will study occur in solution, so we will deal with concentrations (in mol/L or mmol/mL = M Lets consider how the concentration of reactants and products change as a function of time. Depending on the extent to which a reaction is reversible, 4 different scenarios can be imaged:  

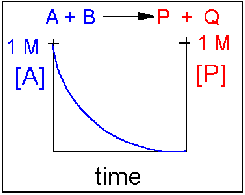
Scenario 1: Irreversible reaction in which the reverse reaction occurs to a negligible extent.

In this reaction, the reverse reaction occurs to such a small extent that we can neglect it. The only reaction that occurs is the conversion of reactants to product. Hence all the reactants are converted to product. At equilibrium [A] = 0. Since 1 mol of A reacted, it must form 1 mol of P and 1 mol Q - i.e. the concentration of products at equilibrium is 1 M. At an earlier time of the reaction, (let's pick a time when [A] = 0.8 M), only part of the reactants have reacted (in this case 0.2 M), producing an equal amount of products, P and Q. Graphs of [A] and [P] as a function of timer are shown below. A decreases in a nonlinear fashion to 0 M while P increases in a reciprocal fashion to 1 M concentration. This is illustrated in the graph below.

Examples of irreversible reactions are reactions of strong acids (like nitric, sulfuric, hydrochloric) with bases like OH^- and water, or combustion reactions like the burning of sugars (like trees) and hydrocarbons (like octane) to form CO₂ and H₂O.

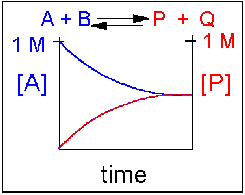
Scenario 2: Reversible reaction in which the forward reaction is favored.

Again [A] decreases and [P] increases, but in this case, some A remains since the reaction is reversible. As A and B decrease, P and Q increase, which increases the chance that they will collide and form product. Since P and Q can react to from reactants, the [A] at equilibriumis not zero as is show below.

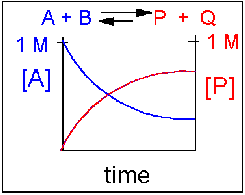
Scenario 3: Reversible Reaction in which forward and reverse reactions are equally favored.

Again [A] decreases and [P] increases, but in this case, some A remains since the reaction is reversible. As A and B decrease, P and Q increase, which increases the chance that they will collide and form product. Since P and Q can react to from reactants, the [A] at equilibrium is not zero as is show below. Because the reactants and products are equally favored, their concentration will be equal at equilibrium.

Scenario 4: Reversible Reaction in which the reverse reaction is favored.

Again [A] decreases and [P] increases, but in this case, some A remains since the reaction is reversible. As A and B decrease, P and Q increase, which increases the chance that they will collide and form product. Since P and Q can react to from reactants, the [A] at equilibrium is not zero as is show below. Because the reaction favors reactants. their concentration will be higher at equilibrium than the products.

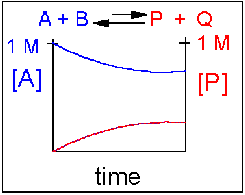
An example of this kind of reaction, one that favors reactants, is the reaction of acetic acid (a weak acid) with water.

Equilibrium Constants:

Without a lot of experience in chemistry, it is difficult to just look at the reactants and products and determine whether the reaction is irreversible, or reversible, favoring either reactants or products (with the exception of obvious irreversible reactions described above). However this data can be found in table of equilibrium constants found in reference books. Look at scenario 2 (a product favored reversible reaction). Consider A <--> P, where at t = 0, A = 1M and P=0M. At equilibrium,  A  might = 0.2 M implying that 0.8 mol/L A reacted forming 0.8 mol/L of P. 80% of A reacted. If initially A = 0.1 M, then at equilibrium,  A = 0.02M and P = 0.08 M. Again, 80% of A reacted. The equilibrium constant, K_eq, for this reaction is: K_eq = [P]_eq/[A]_eq = 0.8/0.2 = 0.08/0.02 = 4. The equilibrium constant, as its name implies, is constant, independent of the concentration of the reactants and products. A K_eq > 1 implies that the products are favored. A K_eq < 1 implies that reactants are favored. When K_eq = 1, both reactants and products are equally favored. For the more general reaction,

aA + bB <==> pP + qQ, where a, b, c, and d are the stoichimetric coefficients,
K_eq = ([P]^p[Q]^q)/([A]^a[B]^b). For a simple reaction where a, b, p, and q are all 1, then
K_eq = ([P][Q])/([A][B]).

For a irreversible reaction, such as the reaction of a 0.1 M HCl(aq) in water, [HCl]_eq = 0, so you can't calculate a K_eq. However, if we assume the reaction goes in reverse to an almost imperceptible degree, [HCl]_eq might equal 10^-10 M. Hence K_eq >> 1.

In summary, the extend of reactions can vary from completely irreversible (favoring only the products) to reactions that favor the reactants . Our next goal is to understand what controls the extent of a reaction. Our answer will be energy changes. We will see example of these different types of reversible reactions later.