MOLECULAR MECHANICS AND DYNAMICS

04/13/16

D.  Bonded Interaction Energy

The mathematical form of the energy terms varies from force-field to force-field. The more common forms will be described.

Stretching Energy: E_stretch = Σ_bondsk_b (r - r_o)²

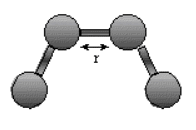
The stretching energy equation is based on Hook's law. The kb parameter defines the stiffness of the bond spring.  R₀ is the equilibrium distance between the two atoms.   It should make sense that deviations from the equilibrium length would be associated with higher energy.  The E vs r curves is hence a parabola:

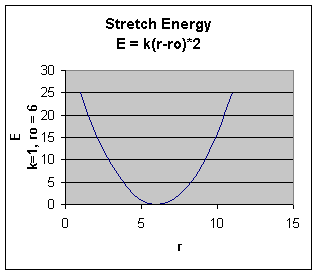
Obviously only small changes in r are allowed as to large an r value would lead to bond breaking.

Bending Energy: E_bending = Σ_angles k_Θ (Θ - Θ_o)²

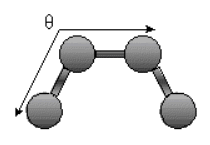The bending energy equation is also based on Hook's law. The kΘ parameter controls the stiffness of the angle spring, while the Θ₀  is the equilibrium angle. As above, the graph of E vs theta is expected to be a parabola.

. 

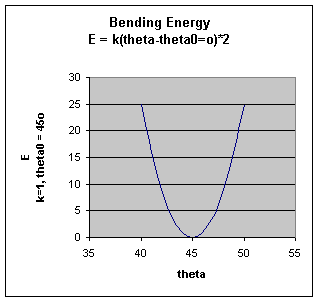
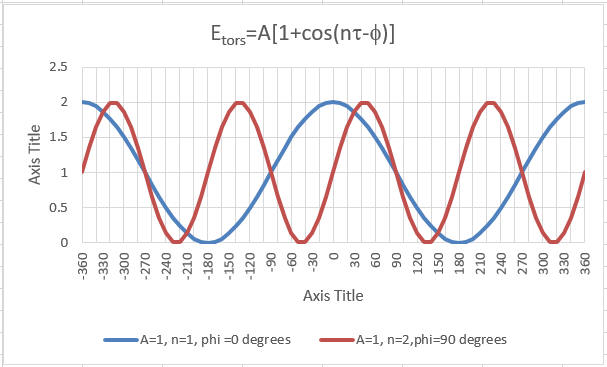
Torsion Energy E_torsion = Σ_torsions A [1 + cos ( ntau - Θ) ] 

The torsion energy is modeled by a periodic function, much as you have seen with energy plots associated with Newman projections sighting down C-C bonds fro butane, for example. 

Wolfram Mathematica CDF Player - Torsional Energy  (free plugin required)

The parameters (determined for different 4 bonded atoms small molecules using curve fitting) for these are:

• amplitude A
• periodicity n (ethane, sighting along the C-C axis in a Newman projects displays a periodicity of 120 degrees)
• phase shift Phi:  shifts curve along rotation (tau) axis.  parameter controls its periodicity, and phi shifts the entire curve along the rotation angle axis (tau).

Interactive SageMath Graph: Interactive Graph of 6-12 Lennard Jones Potential

Navigation

Return to Molecular Mechanics and Dynamics Contents

Return to Biochemistry Online Table of Contents

Archived version:  Molecular Mechanics and Dynamics