Biochemistry Online: An Approach Based on Chemical Logic

CHAPTER 6 - TRANSPORT AND KINETICS
B: Kinetics of Simple and
Enzyme-Catalyzed Reactions
BIOCHEMISTRY - DR. JAKUBOWSKI
4/10/16
|
Learning Goals/Objectives for Chapter 6B: After class and this reading, students will be able to
|
B4. Steady State Enzyme-Catalyzed Reactions
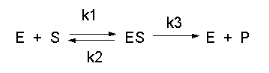
In this derivation, we will consider the following equations and all the rate constants, and will not arbitrarily assume that k2 >> k3. We will still assume that S >> Eo and that Po = 0. An added assumption, however, is that dES/dt is approximately 0. Look at this assumption this way. When an excess of S is added to E, ES is formed. In the rapid equilibrium assumption, we assumed that it would fall back to E + S faster than it would go onto product. In this case, we will assume that it might go on to product either less or more quickly than it will fall back to E + S. In either case, a steady state concentration of ES arises within a few milliseconds, whose concentration does not change significantly during the initial part of the reaction under which the initial rates are measured. Therefore, dES/dt is about 0. In the rapid equilibrium derivation, we observed that v = k3ES. We then solved for ES using Ks and mass balance of E. In the steady state assumption, the equation v = k3ES still holds, but know we will solve for ES using the steady state assumption that dES/dt =0.
Equation 1) v = k3ES
Equation 6: (steady state) dES/dt = k1(E)(S) - k2(ES) -k3(ES) = 0
k1(E)(S) = (k2 + k3)(ES)
k1(Eo-ES)(S) = (k2 + k3)(ES)
k1(Eo)(S) - k1(ES)(S) = (k2 + k3)(ES)
k1(Eo)(S) = (k2 + k3)(ES) + k1(ES)(S)
k1(Eo)(S) = ES (k2 + k3 + k1S)
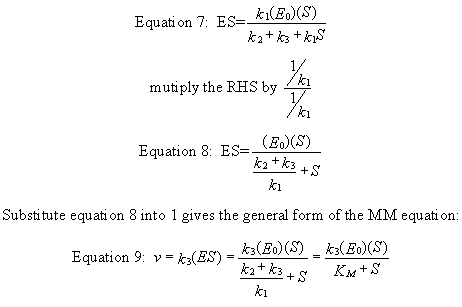
Equation 9) v = k3ES = [k3(Eo)(S)]/ [(k2 + k3)/k1 + S] = [k3(Eo)(S)]/(Km+ S)
Navigation
Return to B: Kinetics of Simple and Enzyme-Catalyzed Reactions Sections
Return to Biochemistry Online Table of Contents
Archived version of full Chapter 5B: Kinetics of Simple and Enzyme-Catalyzed Reactions

Biochemistry Online by Henry Jakubowski is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.